Proving Segments Congruent First

CCSS-M.G-CO.B.8 Explain how the criteria for triangle congruence (ASA, SAS, and SSS) follow from the definition of congruence in terms of rigid motions.

Proving triangle congruence from rigid motions has been one of our most challenging new standards. Which is exciting for me as a teacher, because I’m always up for learning about something that all of the textbooks from which I’ve taught geometry have let slide into our deductive system as postulates with no need of proof.

So after two years of teaching these standards, it occurred to me that maybe we shouldn’t start with proving triangle congruence using rigid motions. Instead, why don’t we see what happens when we start with two segments.

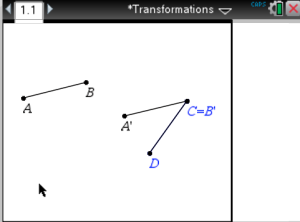
What set of rigid motions will show that segment AB is congruent to segment CD?

Students started creating a plan (sequence of rigid motions) on paper. But before we moved to the technology to test the plans, we talked about attend to precision. Instead of saying that you’ll use a translation and a rotation, let’s be specific about what translation and what rotation. A translation of what segment by what vector? A rotation of what segment about what point using what angle measure?

I began to see the specifics on paper, but while students were pretty confident about the translations they had named, they were not totally confident about the rotations they had named. We needed our technology to help us see.

One team translated segment AB using vector BC.

Then they rotated segment A’B’ about B’ using angle A’B’D.

We can see that it works: the blue pre-image is now black. And we can move the original segment to see that it sticks.

Did this team prove that segment AB is congruent to segment CD? Or did they prove that segment AB is congruent to segment DC? Does it matter?

How many times have we told students that saying segment AB is congruent to segment CD is the same thing as saying that segment AB is congruent to segment DC? It occurred to me in the midst of this lesson that we have actually shown why those endpoints are interchangeable in our congruence statement for segments.

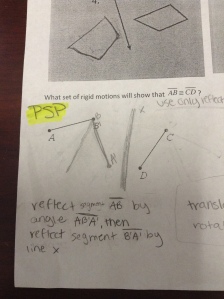
Another team used a translation (segment AB using vector BD) and was trying to use a reflection. When I discussed their work with them, they said, “we know where to draw the line, but we don’t know how to describe it”.

That team presented their work to the class using Live Presenter.

They drew in a line and reflected segment A’B’ about the line.

 

It didn’t work, but they moved the line into the right place.

So what’s significant about the line of reflection that works?

Someone in the class suggested that it’s the angle bisector of angle DCB”.

Is it?

We don’t have to wonder. We can verify using our Angle Bisector tool.

What else is significant about the line?

This year in geometry we are often going to have to see what isn’t pictured (look for and make use of structure).

What if we draw in the auxiliary segment B’D? What else is significant about the line of reflection?

It’s the perpendicular bisector of segment B’D. And again, we don’t have to wonder. We can verify using our Perpendicular Bisector tool.

Can you show that segment AB is congruent to segment CD using only reflections?

We left this exercise for Problem Solving Points, as of course by now we were running out of class time.

One student shared her work with me the next day.

And the next.

We still have work to do. But that’s good … this was the first week of school.

We did this task at my CMC-S session recently, and I asked about using at least one reflection since, like my students, most everyone translated and then rotated.

I was expecting to hear: Reflect segment AB about the perpendicular bisector of segment AC.

Then reflect segment A’B’ about the perpendicular bisector of segment A’D.

Some day I’m going to learn to not be surprised by solutions that are different from mine. One of the participants suggested extending lines AB and CD until they meet at point I. Then reflecting segment AB about the angle bisector of angle BIC. Then they translated segment A’B’ using vector B’C. Several weren’t satisfied with proving segment AB congruent to segment DC, so we noted that we could reflect segment B’’A’’ about its perpendicular bisector to show that segment AB is congruent to segment CD.

 

 

Another participant asked whether students had been confused moving into proving triangles congruent by changing the order of the vertices, since we can’t do that in a congruence statement. We did this at the beginning of the rigid motions unit, and I didn’t notice any issues moving into congruence statements for triangles where the order of the vertices matters.

And so the journey continues … every once in a while figuring out a task that will help us along the way towards meeting our learning goals.